Abstract
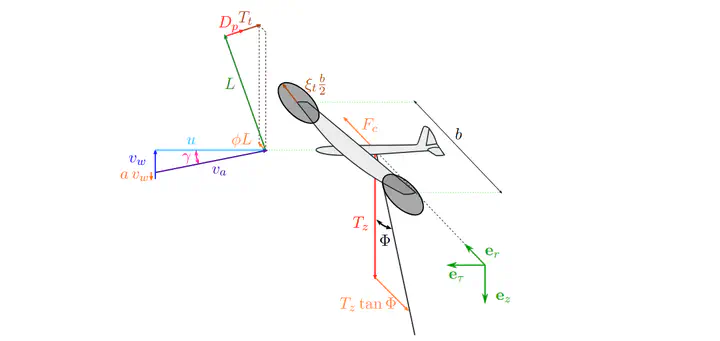
Windplanes (i.e. Fly-Gen airborne wind energy systems) harvest wind power via the turbines placed on the tethered wing, which flies crosswind trajectories. In this paper, the optimal design of windplanes is investigated with simplified models, enabling an intuitive understanding of their physical characteristics. The windplane is then idealized as a point mass flying circular fully crosswind trajectories. If the gravity is neglected, the dynamic problem is axial symmetric and the solution is steady. The generated power can be expressed in non-dimensional form by normalizing it with the wind power passing by a disk with radius the wingspan. Since the reference area is taken to be a function of just the wingspan, looking for the design which maximizes this power coefficient addresses the question ”Given a wingspan, which design maximizes power?”. This is different from the literature, where the design problem is formulated per wing area and not per wingspan. The optimal designs have a finite aspect ratio and operate at the maximum lift-to-drag ratio of the airfoil. Airfoils maximizing the lift-to-drag ratio are then optimal for windplanes. If gravity is included in the model, gravitational potential energy is being exchanged over one revolution. Since this exchange comes with an associated efficiency, the plane mass and the related trajectory radius are designed to reduce the potential energy fluctuating over the loop. However, for decreasing turning radii, the available wind power decreases because the windplane sweeps a lower area. For these two conflicting reasons, the optimal mass is finite.
Alessandro Croce
Associate Professor
My research interest is in multibody-aeroelastic modelling and simulation of wind energy and rotorcraft systems.